Volume & Surface Area.. or, Wrapping Pancakes

We had pancakes tonight. And we had leftovers. So we decided to store them in the fridge to reheat later.

This is the way you are supposed to do it. 

But we just wrap them in foil and stuff them in the fridge. So, as usual, I pulled out a sheet of aluminum foil, eyeballing how much I would need and adding another inch or two for good measure.

Then I stacked the pancakes on top. It looked like this. (Ok so far...)

Two side-by-side stacks, and one extra on top. (That's odd...)

But then I folded up the foil, and that looked like this.

Doesn't fit!

And then I said this:

Doh!

Then I got to thinking: I did something like this with my Math for Elem Teachers students. We were designing a pig pen for Sherman the Pig from the PBS Cyberchase episode Totally Rad, where the number of fence pieces was fixed and they (and we) needed to figure out how to build the pen with the most area for Sherman. I think the activity was one developed (or at least shared) by my colleague Math Hombre.

Oh no, Sherman is stuck!
(Click to open the video in a new window.)

My students' conclusion: in order to get the most area out of the fixed amount of fencing available for Sherman's pen, we need to build it as close to a square in its dimensions as possible.

We were dealing with rectangular regions at the time. We later extended their conjecture when we discovered that there are even better polygons than squares for maximizing area, as long as you don't restrict yourself to quadrilaterals. We decided that the general idea is to make your pen as close to a circle as possible. A square is the most circle-like of the rectangles, so a square pen is better than, say, a long thin rectangular pen.

But of course a hexagon is more like a circle than a square, so that would be a better bet than a square, assuming you don't mind non-square angles.

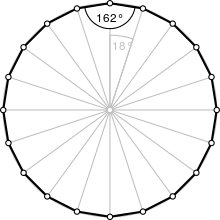
Then again... icosagon, anyone?

Lots of nice extension possible there. But what does all this have to do with wrapping pancakes with not-quite-enough aluminum foil?

I'll let the pictures speak for themselves.
 

Hmm, what if we stack them this way?

Success!

So there you have it. If you make two side-by-side stacks (like a wide rectangle), you don't have enough foil to do the job. But stack them in one tall, more-sphere-like-stack, and the pancakes fits easily inside, and you even have enough spare foil to make a couple of Sherman-the-Pig ears for show.

Woohoo!

Next time I teach Math for Elementary Teachers, there's going to be a new punchline to the old Pig Pen task!